Six Trigonometric Functions

Cotangent Function: f(x)= cot (x)

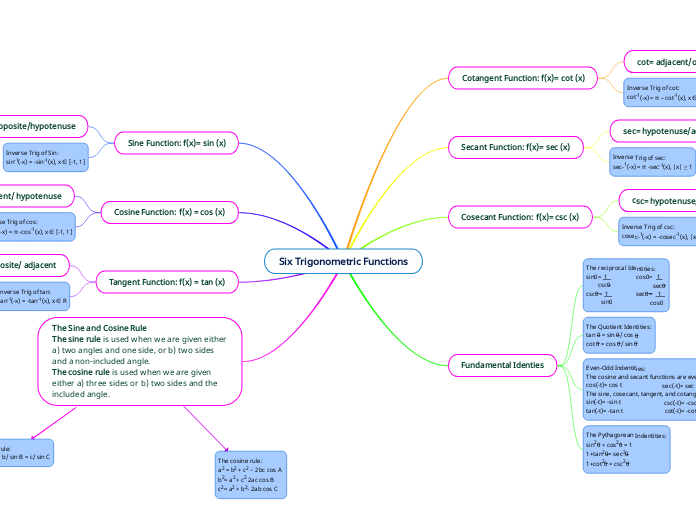
cot= adjacent/opposite

Inverse Trig of cot:
cot-1(-x) = π – cot-1(x), x ∈ R

Secant Function: f(x)= sec (x)

sec= hypotenuse/adjacent

Inverse Trig of sec:
sec-1(-x) = π -sec-1(x), |x| ≥ 1

Cosecant Function: f(x)= csc (x)

csc= hypotenuse/opposite

Inverse Trig of csc:
cosec-1(-x) = -cosec-1(x), |x| ≥ 1

Fundamental Identies

The reciprocal Identities:
sin0= 1 cos0= 1
csc0 sec0
csc0= 1 sec0= 1
sin0 cos0

The Quotient Identities:
tan 0 = sin 0/ cos 0
cot 0 = cos 0/ sin 0

Even-Odd Indentities:
The cosine and secant functions are even.
cos(-t)= cos t sec(-t)= sec t
The sine, cosecant, tangent, and cotangent functions are odd.
sin(-t)= -sin t csc(-t)= -csc t
tan(-t)= -tan t cot(-t)= -cot t

The Pythagorean Indentities:
sin20 + cos20 = 1
1+tan20= sec20
1+cot20 = csc20

Sine Function: f(x)= sin (x)

sin= opposite/hypotenuse

Inverse Trig of Sin:
sin-1(-x) = -sin-1(x), x ∈ [-1, 1]

Cosine Function: f(x) = cos (x)

cos= adjacent/ hypotenuse

Inverse Trig of cos:
cos-1(-x) = π -cos-1(x), x ∈ [-1, 1]

Tangent Function: f(x) = tan (x)

tan= opposite/ adjacent

Inverse Trig of tan:
tan-1(-x) = -tan-1(x), x ∈ R

The Sine and Cosine Rule
The sine rule is used when we are given either a) two angles and one side, or b) two sides and a non-included angle.
The cosine rule is used when we are given either a) three sides or b) two sides and the included angle.

The sine rule:
a/ sin A = b/ sin B = c/ sin C