Geometry
Other Polygons
What is a polygon?
Regular polygons
In a regular polygon, all sides are the same length.(All regular polygon images hosted at http://www.mathleague.com/help/geometry/polygons.htm)

Pentagon

Hexagon

Heptagon

Octagon

Nonagon

Decagon
Irregular polygons
In an irregular polygon, not all sides are the same length.(All irregular polygon images hosted at http://www.mathleague.com/help/geometry/polygons.htm)

Pentagon

Hexagon

Heptagon

Octagon (8 sides)

Nonagon (9 sides)

Decagon
Glossary Page
Triangles
Types of Triangles
Organized by side length
Summary:3 sides the same length: equilateral2 sides the same length: isoscelesNo sides the same length: scalene
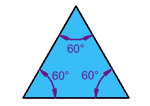
Equilateral
An equilateral triangle has all three sides the same length. Also, all of the angles are 60° angles.(image hosted at http://www.mathsisfun.com/triangle.html)

Isosceles
An isosceles triangle has two sides the same length, and the angles opposite those two sides are also equal.(image hosted at http://www.mathsisfun.com/triangle.html)
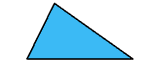
Scalene
A scalene triangle has three sides with different lengths. Also, all three angles have different measures.(image hosted at http://www.mathsisfun.com/triangle.html)
Organized by largest angle
Summary:Largest angle > 90°: ObtuseLargest angle = 90°: RightLargest angle < 90°: Acute

Acute
In an acute triangle, all three angles measure less than 90°.(image hosted at http://www.mathsisfun.com/triangle.html)
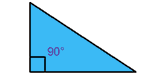
Right
In a right triangle, one angle measures exactly 90°.(image hosted at http://www.mathsisfun.com/triangle.html)
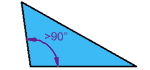
Obtuse
In an obtuse triangle, one of the angles measure more than 90°.(image hosted at http://www.mathsisfun.com/triangle.html)
Special right triangles
Summary: 30/60/90 triangle Side opposite 90°: x Side opposite 60°: x√(3)/2 Side opposite 30°: x/2 45/45/90 triangle Side opposite 90°: x Sides opposite 45°: x√(2)/2
30/60/90
Summary:Length of hypotenuse: xLength of side opposite 30°: x/2Length of side opposite 60°: x√(3)/2 orLength of hypotenuse: 2xLength of side opposite 30°: xLength of side opposite 60°: x√(3)These are equivalent representations, depending on whether x is the short side or the hypotenuse (long side).
45/45/90
Summary:If the length of the hypotenuse is x, the other two sides are of length x√(2)/2.
Area Formulas
There are over 100 formulas for finding the area of a triangle.The three most commonly used ones are:Basic Formula: A = bh/2 b is the base h is the heightUsing Trigonometry: A = ab sin(C) / 2 a is the length of one side b is the length of another side C is the angle between the two known sidesHeron's Formula (also called Hero's Formula): A = square root of: [s(s-a)(s-b)(s-c)] a, b and c are the three side lengths s is the semiperimeter: s = (a+b+c)/2
Basic
Using Trigonometry
Heron's Formula
Other Formulas
Quadrilaterals
Kites

Trapezoids
A trapezoid has one pair of parallel sides.(Image hosted at http://www.mathleague.com/help/geometry/polygons.htm)
Cyclic Quadrilaterals
Glossary Page

Parallelograms
A parallelogram has two pairs of parallel sides. (Image hosted at http://www.mathleague.com/help/geometry/polygons.htm)

Rectangles
A rectangle is a quadrilateral with four 90° angles.(Image hosted at http://www.mathleague.com/help/geometry/polygons.htm)

Squares
A square is a rectangle where all four sides are the same length.(Image hosted at http://www.mathleague.com/help/geometry/polygons.htm)

Rhombuses
A rhombus is a parallelogram where all four sides are the same length.(Image hosted at http://www.mathleague.com/help/geometry/polygons.htm)

Squares
A square is a rectangle where all four sides are the same length.(Image hosted at http://www.mathleague.com/help/geometry/polygons.htm)